Pythagorean Theorem

One of the simplest and most important proofs is the Pythagorean Theorem.  It provides a valuable engineering insight on how to create a right angle using any material to form the angle and a measuring device.  It is named after the legendary Greek mathematician Pythagoras of Samos who according to legend was the first to provide a proof of this theorem.

A right triangle is any triangle where one of its interior angles is 90 degrees. Clearly, there can only be one such angle.  If there were two, then the two opposite lines forming the right angles would be parallel and not be able to form a triangle (this idea is known as the parallel postulate and while it is intuitive, its proof requires some very non-trivial assumptions).

When we look at a right triangle, we can see that the line opposite the right angle is the longest side which is called the hypotenuse.  According to Wikipedia, this name is derived from the Greek for "subtending the right angle".   "Subtending" is a geometric term for forming a side from two lines that meet at a point and form a angle from that point.

The theorem states that for a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other sides.  So, if a triangle has sides of length 3,4, and 5, then it is necessarily a right triangle since the longest side, the hypotenuse, has the following relationship:  5 x 5 = 25 = 3 x 3 + 4 x 4 = 9 + 16.

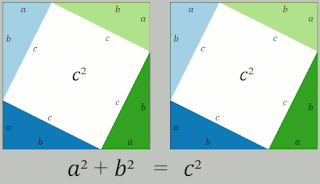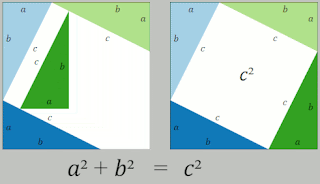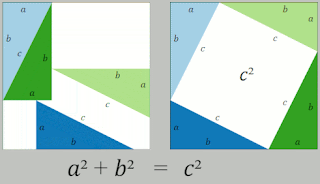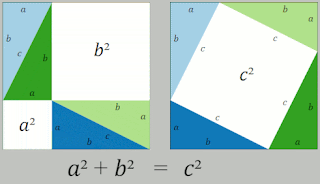
Here is a very simple proof of the Pythagorean Theorem:

The diagram above uses the intuition that a fixed area when moved maintains the fixed area.  The difference between the triangles is always $c^2$ but when the four triangles are moved, the difference is shown to also be the same of $a^2 + b^2$.

Why is this true?  Below in a more detailed argument.

Explanation:

(1)  Let a,b,c be the three sides of any right triangle where c is the hypotenuse.

(2)  If we take four copies of this trial, we can form a square where each side is of length a+b.

(3)  The area of a square is $(a+b)^2 = a^2 + 2ab + b^2$

(4)  The area of a triangle is $\frac{ab}{2}$ since a triangle is exactly half the area of the rectangle $ab$.

(5)  The area of the square is also determined by adding the four triangles with the area of the square in the middle so that:  $(a+b)^2 = 4*(\frac{ab}{2} + c^2

(6)  This suggests that $c^2 = a^2 + 2ab + b^2 - 2ab = a^2 + b^2$

Not only is every right triangle characterized by this equation, it also follows that every triangle whose sides have these ratios is necessarily a right triangle (this follows from the observation that any such triangle must necessarily be congruent to a right triangle).